Multiplication of Fractional Number by a Whole Number

This topic deals with multiplication of a whole number i.e. (0, 1, 2, 3, 4, 5, 6, 7…….) by a fraction i.e. a number in \(\frac{p}{q}\) form. When we are multiplying a whole number with a fraction or fractional number then first we have to change that whole number into fraction just by placing a 1 in the denominator. As 1 in the denominator has no value hence the actual value of the whole number will remain same if we place 1 in the denominator. 

Solved Examples on Multiplication of Fractional Number by a Whole Number:

\(\frac{8}{10}\) × 5

In this sum 5 is the whole number and 8/10 is the fraction. 

Step I: Change the whole number into a fraction by placing 1 in the denominator.

Therefore, \(\frac{8}{10}\) × \(\frac{5}{1}\)

Step II: Now both the numbers are in fraction and the rule of multiplication of fractional number is that numerator and numerator should be multiplied and similarly denominator and denominator should be multiplied. So, we will do

5 × 8 = 40

And 1 × 10 = 10

Therefore, \(\frac{5}{1}\) × \(\frac{8}{10}\)  = 40/10

Step III: After multiplying numerator by numerator and denominator by denominator the answer that we will get has to be either changed into lowest term (if possible) and then change into mixed fraction if not a proper fraction or can be left as whole number (if possible).

Therefore, first we will change 40/10 into lowest term

= \(\frac{40 ÷ 10}{10 ÷ 10}\)

= \(\frac{4}{1}\)

= 4  [This can be written as 4 as 1 in the denominator has no value it remains same.] 

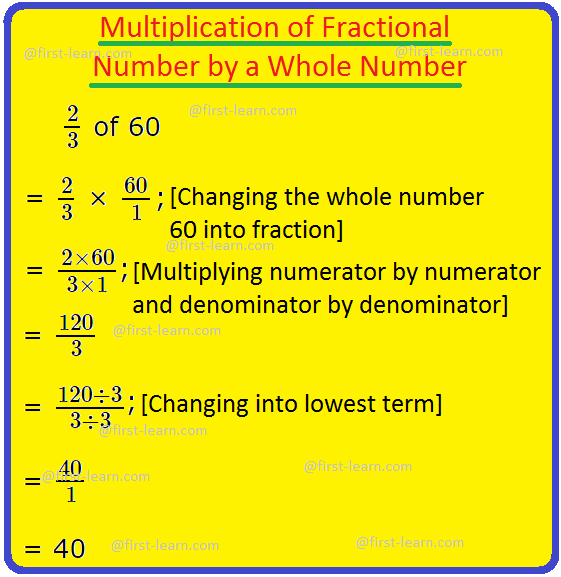
2. \(\frac{2}{3}\) of 60

Here, ‘of ‘denotes multiplication, 

So, \(\frac{2}{3}\) × 60

= \(\frac{2}{3}\) × \(\frac{60}{1}\); [Changing the whole number 60 into fraction]

= \(\frac{2 × 60}{3 × 1}\), [Multiplying numerator by numerator and denominator by denominator]

= \(\frac{120}{3}\)

= \(\frac{120 ÷ 3}{3 ÷ 3}\), [Changing into lowest term]

= \(\frac{40}{1}\)

= 40

3. \(\frac{7}{54}\) × 3

= \(\frac{7}{54}\)  × \(\frac{3}{1}\); [Changing the whole number 60 into fraction]

= \(\frac{7 × 3}{54 × 1}\); [Multiplying numerator by numerator and denominator by denominator]

= \(\frac{21}{54}\)

= \(\frac{21 ÷ 3}{54 ÷ 3}\); [Changing into lowest term]

= \(\frac{7}{18}\); [Cannot be changed into mixed fraction as it is a proper fraction]

4. 4\(\frac{3}{15}\) × 10

= \(\frac{15 × 4 + 3}{15}\) × 10; [Changing improper fraction into mixed fraction]

= \(\frac{63}{15}\) × 10

= \(\frac{63}{15}\) × \(\frac{10}{1}\); [Changing the whole number 10 into fraction]

= \(\frac{63 × 10}{15 × 1}\); [Multiplying numerator by numerator and denominator by denominator]

= \(\frac{630}{15}\)

= \(\frac{630 ÷ 15}{15 ÷ 15}\); [Changing into lowest term]

= \(\frac{42}{1}\)

= 42

5. \(\frac{2}{4}\) × 15

= \(\frac{2}{4}\) × \(\frac{15}{1}\); [Changing the whole number 10 into fraction]

= \(\frac{2 × 15}{4 × 1}\); [Multiplying numerator by numerator and denominator by denominator]

= \(\frac{30}{4}\)

= \(\frac{30 ÷ 2}{4 ÷ 2}\); [Changing into lowest term]

= \(\frac{15}{2}\); [Changing improper fraction into mixed fraction]

= 7\(\frac{1}{2}\); [Answer is expressed in mixed fraction as it was an improper fraction in the 2nd last step]

Hence, these are the various types of sums where we are multiplying a whole number with a fraction.

From Multiplication is Repeated Addition to HOME PAGE