Properties of Division

There are few properties of division like other basic operations (such as addition, subtraction and multiplication) which are helpful in better understanding of the concept and ideas. These properties will help the learners in easier understanding and even for the purpose of self checking the division problems done. Each of the properties is narrated below:

1. If any whole number (other than zero) is divided by itself then the answer or the quotient is one.

For Example:

(i) 5 ÷ 5 = 1

Again, as we know that division is an inverse of multiplication hence 5 = 5 × 1

(ii) 25 ÷ 25 = 1

Again, as we know that division is an inverse of multiplication hence 25 = 25 × 1

2. If any whole number (other than zero) is divided by 0 then it is undefined or meaningless

For Example:

(i) 47 ÷ 0 = undefined

(ii) 5 ÷ 0 = undefined

(iii) 225 ÷ 0 = undefined

3. If zero divided by a non- zero whole number, the answer is zero.

For Example:

(i) 0 ÷ 5 = 0

(ii) 0 ÷ 10 = 0

(iii) 0 ÷ 50 = 0

4. If any non zero whole number is divide by 1, then the quotient will be the whole number itself.

For Example:

(i) 5 ÷ 1 = 5

(ii) 23 ÷ 1 = 23

(iii) 15 ÷ 1 = 15

5. If any whole number (other than zero) is divided by another non-zero whole number, the quotient is not necessarily a whole number.

For Example:

(i) 25 ÷ 2 = 12.5

(ii) 10 ÷ 3 = 3.333

(iii) 27 ÷ 4 = 6.75

So, in these cases we can see that a whole number is divided by another whole number and the result is not a whole number. The answer is in decimal.

6. If there are three whole number out of the three one is dividend, another divisor and other is quotient. Now if the divisor and the dividend is not zero then divisor multiplied by quotient is dividend.

For Example:

(i) 25 ÷ 5 = 3

Here, 25 is the dividend, 5 is the divisor and 3 is the quotient.

Now according to the property divisor multiplied by quotient is the dividend

So, 5 × 3 = 15

(ii) 50 ÷ 10 = 5

Here, 50 is the dividend, 10 is the divisor and 5 is the quotient.

Now according to the property divisor multiplied by quotient is the dividend

So, 5 × 10 = 50

iii) 39 ÷ 13 = 3

Here, 39 is the dividend, 13 is the divisor and 3 is the quotient.

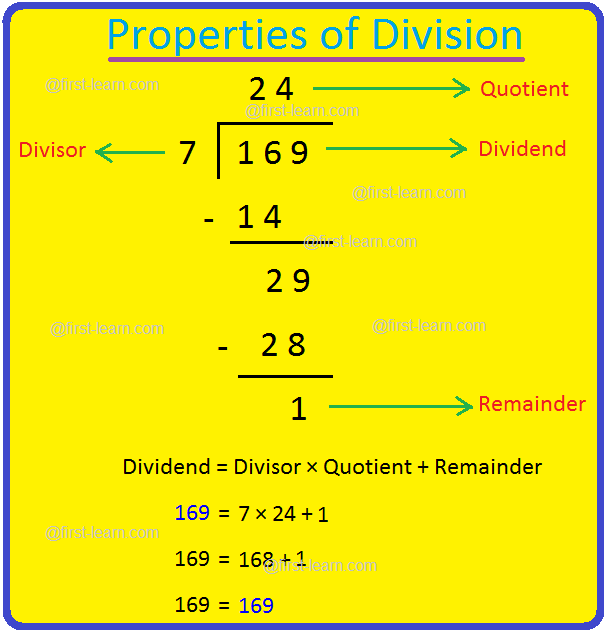
Division Equation:  Dividend = Divisor × Quotient + Remainder

 

Now according to the property divisor multiplied by quotient is the dividend

So, 13 × 3 = 39

169 ÷ 7

Dividend = Divisor × Quotient + Remainder

             = 7 × 24 + 1

             = 168 + 1

             = 169

7. If there are three whole numbers (other than zero) p, q and r. If q × r = p, then p ÷ r = q and p ÷ q =r

For Example:

(i) 8 × 6 =48

Then, 48 ÷ 6 = 8 and

48 ÷ 8 = 6

(ii) 9 × 5 = 45

Then, 45 ÷ 5 =9 and

45 ÷ 9 = 5

From Properties of Division to HOME PAGE